General
The rational method is used to predict peak flows for small drainage areas which can be either natural or developed. The rational method can be used for culvert design, pavement drainage design, storm drain design, and some stormwater facility design. The greatest accuracy is obtained for areas smaller than 40 hectares (100 acres) and for developed conditions with large areas of impervious surface (e.g., pavement, roof tops, etc.). Basins up to 400 hectares (1,000 acres) may be evaluated using the rational formula; however, results for large basins often do not properly account for effects of infiltration and thus are less accurate. Designers should never perform a rational method analysis on a basin that is larger than the lower limit specified for the USGS regression equations since the USGS regression equations will yield a more accurate flow prediction for that size of basin.
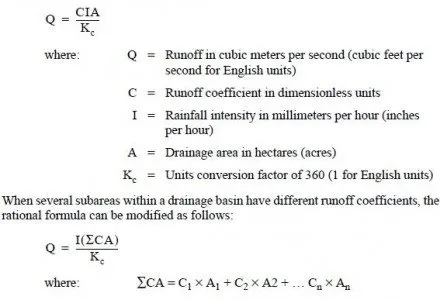
The formula for the rational method is:
Hydrologic information calculated by the rational method should be submitted on DOT Form 235-009 (see Figure 2-4.1). This format contains all the required input information as well as the resulting discharge. The description of each area should be identified by name or stationing so that the reviewer may easily locate each area.
The runoff coefficient C represents the percentage of rainfall that becomes runoff. The rational method implies that this ratio is fixed for a given drainage basin. In reality, the coefficient may vary with respect to prior wetting and seasonal conditions. The use of an average coefficient for various surface types is quite common and it is assumed to stay constant through the duration of the rain storm.
Frozen ground can cause a dramatic increase in the runoff coefficient. When this condition is coupled with heavy rainfall and, perhaps, melting snow, the runoff can be much greater than calculated values that did not account for these conditions. This condition is common for larger basins that are above 300 m (1000 ft.) in elevation and is automatically accounted for in the USGS regression equations. For small basins where the rational method is being used, the designer should increase the runoff coefficient to reflect the reduction in infiltration and resulting increased surface runoff.
In a high growth rate area, runoff factors should be projected that will be characteristic of developed conditions 20 years after construction of the project. Even though local storm water practices (where they exist) may reduce potential increases in runoff, prudent engineering should still make allowances for predictable growth patterns.
The coefficients in Figure 2-4.2 are applicable for peak storms of 10-year frequency. Less frequent, higher intensity storms will require the use of higher coefficients because infiltration and other losses have a proportionally smaller effect on runoff. Generally, when designing for a 25-year frequency, the coefficient should be increased by 10 percent; when designing for a 50-year frequency, the coefficient should be increased by 20 percent; and when designing for a 100-year frequency, the coefficient should be increased by 25 percent. The runoff coefficient should never be increased above 0.90.
Hydrologic information calculated by the rational method should be submitted on DOT Form 235-009 (see Figure 2-4.1). This format contains all the required input information as well as the resulting discharge. The description of each area should be identified by name or stationing so that the reviewer may easily locate each area.